Checkerboard Patterns with Black Rectangles

Abstract

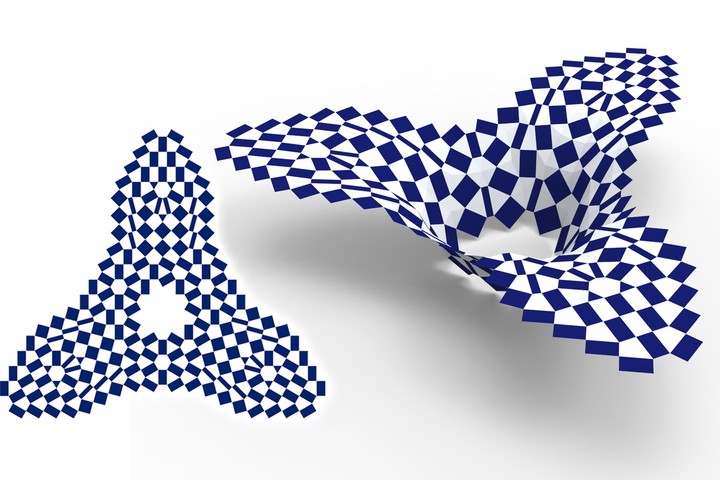
Checkerboard patterns with black rectangles can be derived from quad meshes with orthogonal diagonals. First, we present an initial theoretical analysis of these quad meshes. The analysis reveals many possible applications in geometry processing and also motivates the numerical optimization for aesthetic and functional checkerboard pattern design. Second, we describe an optimization algorithm that transforms initial 2D and 3D quad meshes into quad meshes with orthogonal diagonals. Third, we present a 2D checkerboard pattern design framework based on integer programming inspired by the logo design of the 2020 Olympic games. Our results show a variety of 2D and 3D checkerboard patterns that can be derived from 2D or 3D quad meshes with orthogonal diagonals.