Shape-morphing Mechanical Metamaterials

Abstract

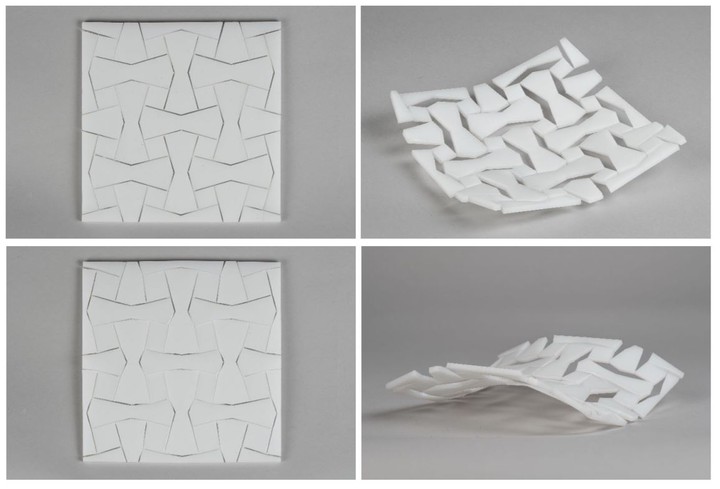
Small-scale cut and fold patterns imposed on sheet material enable its morphing into three-dimensional shapes. This manufacturing paradigm has been receiving much attention in recent years and poses challenges in both fabrication and computation. It is intimately connected with the interpretation of patterned sheets as mechanical metamaterials, typically of negative Poisson ratio. We here present an affirmative solution to a fundamental geometric question, namely the targeted programming of a shape morph. We use optimization to compute kirigami patterns that realize a morph between arbitrary shapes, in particular between a flat sheet and a surface in space. This so-called inverse problem for kirigami cut and fold patterns is solved by drawing on a differential-geometric interpretation of the morph and on progress in geometric computing